Lattice Energy

Lattice Energy Definition

Ionic compounds are more stable because of their elctrostatic force between the two opposite ions. After the formation of ions, they combine together to form ionic compound. The energy released in this process is known as lattice energy or lattice enthalpy. That means, energy released when a cation and a anion combine together to form one mole of an ionic compound is know as lattice energy or lattice enthalpy. Thus, we can write

A⁺ + B^– → A⁺B^– + Lattice energy

Factors that affect lattice energy

The strength of ionic bond increases with the increase of lattice energy. And lattice energy depends on two factors: size or radius of ions and charge of ions.

a) Radius of ions

As the radius of ions increases, the lattice energy decrease. Below is a graph of the lattice energy of lithium halide. As the size of halide increases down the group, the lattice energy decreases.

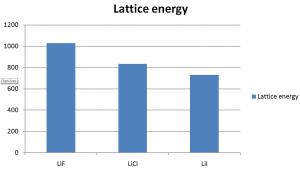
This is because with the increase of size of ions, the distance between their nuclei increases. Thus the attraction between them decreases and finally the less lattice energy released during the process.

b) Charge of ions

Lattice energy increases with increase of charge on the ions because of their more attractive force between them. Thus +2 or -2 ions will release more lattice energy than the +1 or -1 ions.

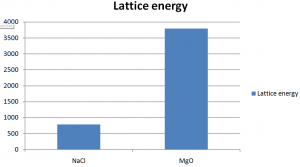
Here we can see that the lattice energy of MgO is much greater than the lattice energy of NaCl.

Calculation of lattice energy

The calculation of lattice energy can be done by using Hess’s law (for this case it is called Born Haber cycle). According to this law the energy change is same for a particular reaction regardless whether the reaction undergoes in one or several steps. So the total energy involved to form a ionic compound from its elements will be same whether it happened in few steps or in one step. For an example, the solid sodium and chlorine gas reacts to form sodium chloride crysltal. The energy change will be same whether it reacts in few steps or in one step. Below is the Born haber cycle for the formation of sodium chloride crystal.

Here the formation of sodium chloride is happening is few steps:

Step 1:

First step involved the formation of sodium atom in gaseous state from its standard solid sodium.

Na_(s) → Na_(g)    ΔH_atom = +107 kJ/mol

This is known as atomisation and the energy change during this process is knows as atomisation energy. The energy change involved to get 1 mole of gaseous atom from its standard state is known as atomisation energy. The atomisation energy for this step is +107 kJ/mol.

Step 2:

Second step involved the dissociation of chlorine molecule to form chlorine atoms in gaseous state. The energy of dissociation of bond energy is +122 kJ/mol.

1/2Cl_2(g) → Cl_(g)    ΔH_BE = +122 kJ/mol

Step 3:

Third step involved ionisation of sodium in gaseous state to get positively charged sodium ion by losing an electron. The ionisation energy for this step is +494 kJ/mol.

Na_(g) → Na⁺_(g) + e^–   ΔH_IE1 = +494 kJ/mol

Step 4:

The fourth step is the addition of electron to the chlorine atom to get negatively charged chloride ion in gaseous state. The electron released for this process is the electron affinity and for this it is -349 kJ/mol.

Cl_(g) + e^– → Cl^–_(g)   ΔH_EA1 = -349 kJ/mol

Step 5:

Fifth and the last step is the formation of sodium chloride from it gaseous ions. The lattice energy released in this process should be negative and need to be calculated.

Na⁺_(g) + Cl^–_(g) → NaCl_(s)  ΔH_LE = -? kJ/mol

The heat of formation for the whole reaction if it occurs in one step is -411 kJ/mol.

Na_(s) + 1/2Cl_2(g) → NaCl_(s)  ΔH_f = -411 kJ/mol

According to Hess’s law

ΔH_f = ΔH_atom + ΔH_BE + ΔH_IE1 + ΔH_EA1 + ΔH_LE

⇒ -411 = 107 + 122 + 494 -349 + ΔH_LE

⇒ ΔH_LE = -785 kJ/mol

So, the lattice energy of sodium chloride is -785 kJ/mol. Similarly lattice energy of any ionic compound can be calculated.

4.56/5 (32)